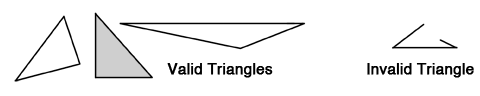
#GRE Geometry - Triangles

A triangle is a 3-sided figure that is drawn using exactly three points and 3 lines, and a triangle is named according to its vertices. The three lines which make up the triangle are called the sides of the triangle, and they can be of different lengths, but the sum of the lengths of the two shorter sides of a triangle cannot be less than the longest side of the triangle, or else the triangle is not closed. Shown below are several triangles, as well as a shape that is not a triangle, because the sum of the two smaller sides is less than the length of the longest side.

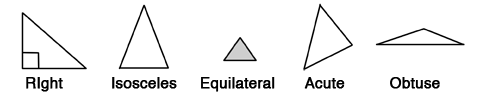
By definition, the sum of the angles of a triangle ALWAYS add up to 180 degrees. Also, certain special triangles have unique names.

• A right triangle is a triangle that has an angle of 90 degrees
• An isosceles triangle has two sides of equal length
• An equilateral triangle has three sides of equal length
• An acute triangle is one where all of the angles are less than 90 degrees
• An obtuse triangle is a triangle where there is one angle that is more than 90 degrees.

Shown below are several triangles:

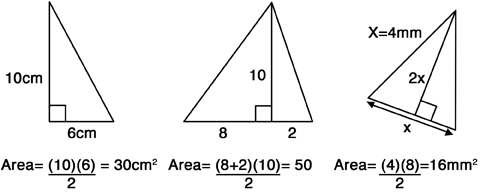
Area of a Triangle

The area of a triangle is one-half the base times height, or, as more commonly written, (1/2)bh. where b designates the length of the base and h designates the height. The height of the triangle, h. is always perpendicular to the base. Here are several triangles and their bases, heights, and areas indicated.

In the left-most triangle, you are explicitly given the base and the height, and so to arrive at the answer you multiply the two numbers and divide by two. In the center triangle, the area of the entire triangle is the total length of the base, which is 8 plus 2, times the height, and all divided by 2 to get 50. In the right-most triangle, note that the "base" does not need to be at the bottom of the picture; it's only important that the height segment be perpendicular to the base. Also, in the right-most triangle, x is denoted as 4mm, so plugging in the values of x and using the formula, you can quickly arrive at the area of the triangle.

Similar Triangles

Two triangles, A and B. are similar if they have the same shape, but have different sizes. For example, if triangle A has side lengths 3, 4, and 5 cm, and triangle B has side lengths of 6, 8 and 10cm, then the triangles are similar. Note that two triangles can be similar even if one is rotated or is a mirror image of the other. There are several properties of similar triangles that you should remember:

• Corresponding angles are the same.
• Corresponding sides are in the same proportion.
• Corresponding medians are all in the same ratio.
• Corresponding altitudes are all in the same ratio.
• The ratio of their areas is the square of the ratio of their sides. For example, if triangle X has sides of lengths 3, 4, and 5cm, and triangle Y has sides of lengths 9, 12, and 15cm, then the ratio of their sides is 3:1, and so the ratio of the areas if (3 2 ):(1 2 ) = 9:1.